由于配置出色��,HTC的新機One X和One S的到來之日也備受期待����。本月初英國運營商曾開啟了這兩款新機的網(wǎng)上預(yù)訂業(yè)務(wù)���,而日前,有消息顯示���,這兩款One系列新機將會在下月初到來�。據(jù)英國
2012-03-19 10:43:18 809
809 按照相關(guān)說法��,HTC One X港行版將在四月二日舉辦的發(fā)布會上正式面市�,屆時還有HTC One系列新機HTC One V同步登場。
2012-03-28 09:34:51 1126
1126 作為HTC旗下最新的“HTC One系列”新機�,最近在媒體上我們見到的皆是HTC One X和HTC One V 即將上市的消息,讓人似乎遺忘了另一款超薄機型HTC One S 的存在
2012-03-30 09:24:43 894
894 第一次嘗試12.1����。我復(fù)制了一個現(xiàn)有的11.4目錄并為12.1創(chuàng)建了一個新的導(dǎo)入/轉(zhuǎn)換項目目錄。 11.4在10分鐘內(nèi)完成建造(目標(biāo)XC3S400A)���。 12.1似乎忽略.ucf文件(如果我在其
2018-10-23 10:25:36
resistor in place and just run a jumper wire from a spare microcontroller pin to the WP pin
2019-01-29 09:16:25
I measured the reading rate, it takes ~60ms to perform one reading. This seems to be much longer
2019-05-30 15:00:11
42841A Resistor Box Set(PN 42841-65002)服務(wù)手冊
2019-04-26 14:07:17
FROM-TOEditor的應(yīng)用
2019-06-26 22:23:40
嗨��,我使用S29 GL001GS并行NOR Flash��,我所面臨的問題是我不能寫完整的1GbT����,我只能寫512MbT��,地址映射是從0x000到0x3ffffFF�����,只有512MbT��,數(shù)據(jù)表指定它是16
2018-12-06 14:55:29
requirement that the power drawn from each phase must be the same. This article discusses one way
2016-06-12 09:26:32
FLIR ONE PRO-IOS
2023-03-28 13:15:52
Chris Johnson Jersey one solid image on the non-address side of the postcard????????? Promise A
2010-11-17 10:24:59
in the path the voltage takes during the integration, as this resistor is actually a thermistor, which
2019-03-08 06:21:27
schematic and LTspice simulation) if there is also a resistor to ground from the ZCDxINpin? (R5
2019-02-15 13:12:49
The handheld that takes 2 Mb/s testing beyond convention
2019-06-17 16:59:59
series diodes from the sense resistor to the RS+ and RS- pins. During normal operating conditions
2011-08-21 16:49:34
to program STM8S timer1 in One Pulse mode, after reading the data-sheet of STM8S003F3P6,I have
2019-01-08 15:41:32
Single-Ended and Differential S-ParametersMAXIM High-Frequency/Fiber Communications GroupThe term
2008-07-23 12:34:04
in an S6LX45. It uses one PLL and eight DCMs. The eight DCM clock inputs each come from a GCLK pin feeding a
2019-07-26 13:04:49
V-by-One是專門面向圖像傳輸開發(fā)出的數(shù)字接口標(biāo)準(zhǔn)����。信號的輸入輸出水平采用LVDS(低電壓差動信號)。板卡的信號頻率約為1GHz�。與此前的CMOS/TTL方式相比,可將傳輸線的數(shù)量減少至此
2013-03-14 18:05:56
目前接口分析:1.TTL:局限性太大���,一般在小屏上面應(yīng)用較多(7寸以下)2.7寸以上的屏現(xiàn)在的接口有LVDS,eDP,iDP,V-BY-ONE�����。附件講述一下由THine公司發(fā)布的V-BY-ONE接口的技術(shù)優(yōu)勢��。需要詳細資料的請電話聯(lián)系�����。
2013-03-14 18:24:27
親愛的先生�����,V-by-One發(fā)射器已經(jīng)發(fā)送了D10.2用于CDR�,但我無法在GTX接收器上讀取D10.2�����。 RXCDRLOCK已經(jīng)輸出高電平。 UG476表示RXCDR_CFG是協(xié)議特定的���,但沒有
2020-08-07 09:03:54
met one 粒子計數(shù)器4800資料
2014-11-05 16:32:55
有哪位能指點一下 怎么設(shè)置non-ideal-resistor里面的“value”選項卡下面的“model type”當(dāng)設(shè)置為 “capacitance=f(voltage)”下面的”table“ 具體應(yīng)該怎么設(shè)置啊謝謝了
2015-02-11 05:04:24
When used as a zenerdiode/resistor combination replacement, the LM78LXX usuallyresults
2008-09-08 11:30:14
;Failed to read from DHT sensor!\"));
return;
}
// Compute heat index in Fahrenheit (the default
2023-05-25 06:21:26
This application note takes you through the process of replacing one instrument in a typical GPIB
2019-10-22 14:55:07
bits of Port A. To limit the current through the LED’s there is one 270 current limiting resistor
2012-08-16 21:09:42
請問�����。在設(shè)計FPGA程序時�����,誰遇到過如下的警告?���。?One or more signals are missing in the process sensitivity list.
2016-05-17 15:57:59
some help. It's mentioned that the sensor need a pull-up resistor but I read the breakout sensor has
2018-08-23 09:34:46
xbox one 解碼The Microsoft Edge browser on Xbox One consoles has a private browsing mode named
2022-01-21 11:57:32
). I want to switch from EP1C12F256C6 to XC3S200AN-FTG256. I've synthesized the RTL codes in ISE
2019-01-30 08:57:42
and each channels have at least two S21 traces (one phase, another log). But when I set up
2018-11-19 10:57:51
such as MCP4261. how can it be determined from the datasheet, how long it takes for the resistance
2019-03-07 13:18:24
on the picture below. Each entity takes another entity ouput as input data, but is operating on clock's
2019-03-26 09:23:58
, this needs to feed in to one of our BT modules based on the 20719, which takes this stream and transmits
2018-09-26 16:41:22
```電阻選型你踩了多少坑�?上睿思電阻商城,輕松不燒腦�����,工作放松兩不誤�����。Resistor Today睿思電阻商城全新改版上線���,新會員***次抽獎機會�����,100%中獎,15萬會員抽獎樂此不疲�����。會員福利
2020-03-31 12:10:17
is that it takes too long time to return from endpoint reset function. I only changged board firmware
2019-05-24 09:48:01
; constraints to prevent 10 signals from removing from Synthesis, but one signal is still missing
2019-02-26 09:15:05
to one以后��,參考Z-stack Developer's guide進行過程分析,請問5.4.3的Route Record Command需要我們自己配置么���,還是在發(fā)送DATA的時候底層自己就發(fā)送
2018-08-09 09:05:51
, implementing(...)主要特色Operates at voltage ranging from 6V to 42V (10s li-ion battery) delivering 25ARMS
2018-09-29 09:34:39
請教一下各位大佬�����,針對IO配置寄存器Default setting takes place at power-up only的疑問���?只能在上電的時候復(fù)位成默認值?那如果我在初始化時候配置錯了��,導(dǎo)致3914工作不正常�,后面我在沒有斷電的情況下重新初始化還可以改過來嗎?
2023-08-07 06:55:01
target is a 2s4p (two LEDs in series and four in parallel) configuration of eight LT W5SG LEDs from OSRAM
2014-01-24 14:46:15
Resistor Color Coder is free, and is very useful for determining the color codes or values
2008-05-09 19:09:47 38
38 of enhancedpackages. One with high popularity is the TO-220 packagedesigned with exposed heat sink for efficient heat dissipation.
2008-06-17 11:36:14 228
228 has one 3-terminal potentiometer and one 2-terminal variable resistor (Figure 1). The MAX5403/MAX5404/MAX5405 operate from +2.7V to +5.5V sing
2008-10-04 20:01:18 17
17 The consideration about “heat” in PCB design
The consideration of EMC, thermal conduct
2009-03-24 14:05:52 0
0 translator to go from a lower to higher voltage and a second one to go from a lower voltage to a higher voltage. This note will outline one pos
2009-05-27 12:58:24 13
13 Systemc From The Ground Up:The first question any reader should ask is “Why this book?” We
2009-07-10 17:27:57 0
0 The AD22105 is a solid state thermostatic switch. Requiring only one external programming resistor
2009-08-23 20:59:18 14
14 The AD622 is a low cost, moderately accurate instrumentation amplifier that requires only one
2009-08-27 10:12:00 13
13 The AD73761 is one of the few high voltage, high performance digital potentiometers2 on the market.
2009-09-22 08:29:33 10
10 The AD5290 is one of the few high voltage, high performance, and compact digital potentiometers2
2009-09-22 08:47:45 14
14 One IC Generates Three Sub-2V Power Rails from a Li-Ion Cell:Shrinking geometries in IC technology
2009-09-30 19:53:31 11
11 Linux from scratsh新手教程.rar
Linux From Scratch 版本6.2 Gerard Beekmans Copyright 1999–2006 Gerard Beekmans 謹以本書獻給 LinuxSir.org 以及所有熱愛 Linux 的人們�����。
2010-03-10 14:07:55 35
35 the functional differences and the electrical specification differences that are present when migrating from one device to t
2010-05-04 12:06:41 24
24 The TMP300 is a low-power, resistor-programmable,digital output temperature switch. It allows
2010-06-01 08:37:36 33
33 and low dropout voltage. The current is adjustable from 10mA to 400mA with an external resistor. Only one external resistor
2010-06-12 08:54:10 46
46 BITMAP CONVERSION FROM ROWIDS
2010-08-03 14:59:47 5
5 conditions. Based on motor manufacturers’ analysis, about 44% of motor failure problems are related to heat resulting from overload
2010-08-17 10:54:56 5
5
Implementing an Eight-to-One USB Switch for KVM Applications
Abstract: Many servers
2009-03-31 09:48:43 828
828 
from one of several computers to a front panel. This application note focuses on the keyboard/mouse portion, and presents a circuit for impl
2009-04-20 11:00:02 490
490 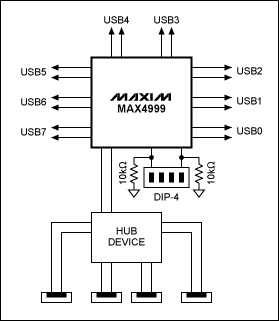
) and mid-range frequency response of a digital potentiometer in VCO control applications by adding a single external resistor between H and W.
2009-04-28 11:43:11 1294
1294 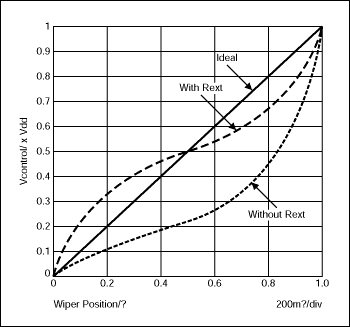
sense resistor to measure current. In certain applications, heat generated by the flow of current through the sense resistor can introduc
2009-04-30 11:58:55 538
538 
高配Aspire one 六芯電池 ——Aspire one Pro 531h
Acer Aspire one 在上網(wǎng)本中一直處于領(lǐng)先的地位�,可想而知Acer在上網(wǎng)本方面下了多大
2009-12-31 08:41:16 644
644 什么是one-stop shopping
英文縮寫: one-stop shopping
中文譯名: 一站購齊
分 類: IP與多媒體
解 釋: 1986年
2010-02-23 10:24:20 879
879 Nexus One是否帶來新的希望���?
谷歌Nexus One智能手機在今年初拉斯維加斯舉行的消費電子展覽會(CES)上首次華麗登場����。上市銷售的Nexus One貼的是谷歌商標(biāo),但在臺灣宏達
2010-03-03 10:25:16 841
841 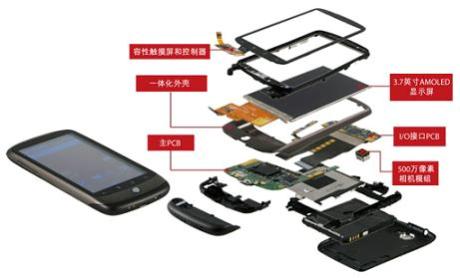
By adding an external resistor network you can lower the gain of a fixed-gain differential
2010-07-18 15:34:44 1061
1061 
This application note shows how a variable resistor controlled by an integrated, programmable
2010-07-18 15:35:44 2368
2368 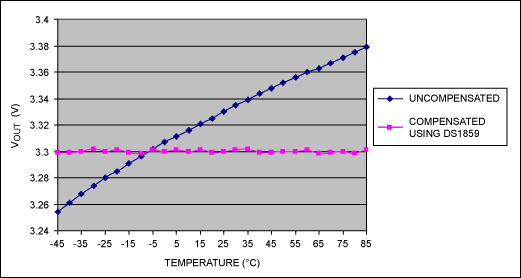
三星i9300和htc one x哪個好,i9300和htc one x的區(qū)別是什么�����?三星i9300相比htc one x哪些好呢?小編在這里為大家總結(jié)了一些三星i9300和htc one x的區(qū)別供大家參考����。
2012-09-14 16:29:27 28474
28474 憑借時尚的設(shè)計和出色的用戶體驗,HTC One已成為今年最流行的 Android終端之一�。而對于喜歡HTC One但又希望尺寸小一點、價格低一點的用戶來說�����,最新發(fā)布的HTC One mini智能手機就是完美之選��,它采用高通驍龍?400系列處理器����,保證高性能的同時,還進行了電池優(yōu)化����。
2013-07-25 11:08:58 779
779 ONE 主板電路圖·能讓你更了解你的手機。沒事看看
2015-12-14 14:08:20 4
4 HackRF One設(shè)計介紹��,支持GNURadio的全開源軟件無線電 10MHz - 6GHz�,包括電路原理圖和pcb文件
2015-12-23 11:07:33 78
78 linkit one 開發(fā)指導(dǎo)書,講解linkit one 開發(fā)板的基礎(chǔ)指導(dǎo)書
2016-02-24 10:27:10 3
3 DSP之run_from_xintf教程�,很好的DSP自學(xué)資料,快來學(xué)習(xí)吧�。
2016-04-15 16:55:30 12
12 Kitchen_DNA_Extraction_from_Strawberries
2017-02-15 23:00:47 0
0 Migrating to Swift from Android
2017-03-19 11:24:33 0
0 Get Fast Stable Response From Improved Unity-Gain Followers
2017-03-24 15:46:21 0
0 Xbox One X和Xbox One S是微軟推出的兩款游戲機�,服務(wù)不同的群體,哪一個適合你���?要做出決定并不像想像的那樣容易���。
2017-06-13 14:53:05 5289
5289 The AD5290 is one of the few high voltage, high performance�����, and compact digital potentiometers2
2017-10-12 13:46:49 18
18 The AD73761 is one of the few high voltage�����, high performance digital potentiometers2 on the market.
2017-10-14 15:02:43 13
13 本文檔內(nèi)容介紹了基于Video-Object-Segmentation-from-MPEG4講解�,供參考
2018-03-15 10:32:55 2
2 最薄手機 HTC One S大拆解
2018-07-06 01:13:00 2974
2974 貼片電阻標(biāo)準(zhǔn)阻值規(guī)格表,Chip resistor
關(guān)鍵字:貼片電阻標(biāo)準(zhǔn)阻值規(guī)格表
貼片電阻標(biāo)準(zhǔn)阻值規(guī)格表
E3
2018-09-20 18:20:32 4662
4662 常用光敏電阻的規(guī)格參數(shù),Photosensitive resistor
關(guān)鍵字:光敏電阻����,元件選擇
規(guī)格
2018-09-20 18:53:58 1344
1344 MEET.ONE 團隊在 DAPP 智能合約開發(fā)���、錢包開發(fā)���、節(jié)點部署等多個方向積累了豐富的行業(yè)與技術(shù)經(jīng)驗。MEET.ONE 側(cè)鏈宗旨在于擴充 EOS 生態(tài)�,降低 EOS 使用門檻和成本,同時賦能側(cè)鏈 DApp 訪問主網(wǎng)資源���。
2019-01-18 11:16:46 1578
1578 
首先�����,技術(shù)參數(shù)不同F(xiàn)LIR ONE Pro擁有更寬的溫度量程能夠測量介于-20°至400°C之間的溫度FLIR ONE Pro支持至多三個點測溫和至多六個溫度感興趣區(qū)域提供更強的隨身圖像分析與報告功能���。
2019-08-15 16:09:51 5941
5941 在CES 2020消費者電子展上����,一加首款概念機Concept One正式發(fā)布。一加Concept One看起來很漂亮���,被邁凱輪超級跑車中所使用的木瓜色皮革所包覆���。
2020-01-08 13:52:44 2732
2732 此前,三星Galaxy已有多款機型推送One UI 3正式版更新�����,包括Galaxy S20�����、S20+����、S20 Ultra等。另外�,三星Galaxy S10e、S10����、S10+、Note10
2021-01-27 10:47:46 5369
5369 LTC6993: One Shot
2021-02-03 16:42:33 10
10 One Hundred And Eighty Decisions
2021-02-04 10:22:10 0
0 2019 年 5 月,理想汽車正式發(fā)布了第一代理想 ONE����,引起了廣泛關(guān)注。自 2019 年 12 月開始交付至今�,理想 ONE 已累計交付了 5 萬 5 千輛。這個數(shù)據(jù)對于一家新勢力造車品牌來說
2021-05-27 14:42:54 3637
3637 Gun Camera - World War II Photography from Allied(嵌入式開發(fā)入門書籍)-gun,camera,allied,war,ii,photography,from,world
2021-07-30 09:47:57 0
0 From additive manufacturing to 3D4D printing 3 Bre(嵌入式開發(fā)師培訓(xùn)班)-From additive manufacturing to 3D4D
2021-07-30 09:49:58 6
6 Biomimetic Medical Materials From Nanotechnology t(嵌入式開發(fā)需要的資料)-Biomimetic Medical Materials From Nanotechnology to 3D Bioprinting by Insup Noh (z-lib.org)
2021-07-30 09:52:55 8
8 C#基礎(chǔ)教程-查詢-復(fù)合from、where子句(嵌入式開發(fā)平臺排行榜)-文檔為C#基礎(chǔ)教程-查詢-復(fù)合from��、where子句總結(jié)文檔��,是一份不錯的參考資料�����,感興趣的可以下載看看�,,��,��,�����,,����,,���,,���,����,����,
2021-08-04 14:53:24 1
1 電子發(fā)燒友網(wǎng)站提供《針對高溫應(yīng)用的苛刻環(huán)境采集終端(HEAT)EVM平臺.zip》資料免費下載
2022-09-07 11:28:00 0
0 FPGA芯片實現(xiàn)V-by-One的收發(fā),同時例化V-by-One 及SerDes IP即可實現(xiàn)V-by-One通信��。由于SerDes和V-by-One是分開的�����,所以頻率可在要求范圍內(nèi)靈活調(diào)整, 同時FPGA內(nèi)部還可以進行OSD疊加等處理并控制其他外設(shè)���,從而充分利用FPGA資源��。
2022-11-18 11:02:36 1462
1462 python指定導(dǎo)入目錄路徑����,稱為包導(dǎo)入。
通過import和from導(dǎo)入包模塊�。
通過**all**指定導(dǎo)入的模塊和導(dǎo)入的模塊屬性。
## 1.1 python包import和from
2023-02-21 14:20:34 927
927
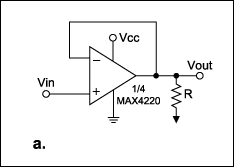
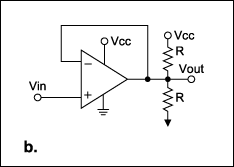
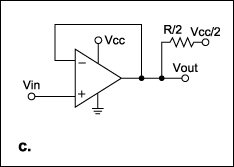


 電子發(fā)燒友App
電子發(fā)燒友App


























評論